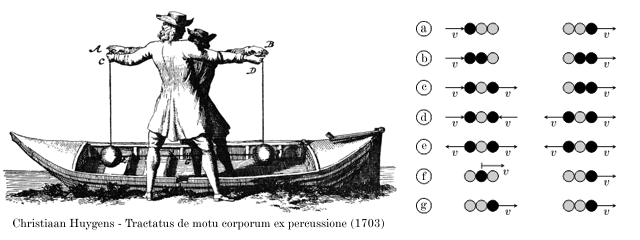
An instantaneous impact law is a crude model of a complex impact process. Existing impact laws, such as Newton’s or Poisson’s impact law, may suffer from non-dissipativity amongst other problems which form real burdens for the simulation of practical application problems. The aim of the PhD project is to divulge the mathematical structure of impact laws using modern concepts from convex and nonsmooth analysis. Fundamental insight may help to remedy the problems of existing impact laws. A major objective of the project is to understand the physical implications of the so-called maximal monotonicity property which the impact law may enjoy. In particular, the question whether this property restricts the set of admissible post-impact velocities is addressed. Furthermore, the project intends to understand under which conditions existing impact laws may lose the property of being maximal monotone. The role of friction in this respect is to be identified. Experimental data will be used to find physical evidence for the maximal monotonicity property of impact laws. The goal is to formulate a parameterized impact law that covers the complete set of possible post-impact velocities while being maximal monotone. Such an impact law will solve the non-dissipativity and related problems of existing impact laws. Moreover, a maximal monotone impact law implies contraction properties which are instrumental for synchronization purposes. The impact law that is to be developed within this project will therefore be of direct application to the control of unilaterally constrained mechanical systems. More specifically, it will allow for the design of synchronization-based state observers for such systems. The project, with its focus on the fundamentals of impact mechanics, will contribute to the emerging research on stability and synchronization theory for nonsmooth dynamical systems.
Publikationen
Book Contributions
Winandy, T., Baumann, M. and Leine, R.I.: "Variational Analysis of Inequality Impact Laws for Perfect Unilateral Constraints", in Advanced Topics in Nonsmooth Dynamics, Eds. R. Leine; V. Acary; O. Brüls, Springer-Verlag, pp. 47-92, 2018.
Journal Publications
Winandy, T. and Leine, R.I.: "A maximal monotone impact law for the 3-ball Newton’s cradle", Multibody System Dynamics, published online 2016. PDF
Proceedings
Winandy, T. and Leine, R.I.: "Towards a Maximal Monotone Impact Law for Newton’s Cradle", in Proceedings of the ECCOMAS Thematic Conference on Multibody Dynamics, Barcelona, Spain, 2015. PDF
Leine, R.I. and Winandy, T.: "Nonexpansivity of the Newton’s Cradle Impact Law", in Proceedings in Applied Mathematics and Mechanics, Vol. 15, pp. 59-60, 2015. PDF
Kontakt

Remco I. Leine
Prof. Dr. ir. habil.Direktor


